Permanent magnet synchronous motor modeling

Classical \(abc\) frame

\[\begin{equation} \label{eq:modeleabc} \left\{\begin{array}{rcl} L \frac{di_{a}}{dt} & = & v_{a}-R i_{a}+ p \phi_f \Omega \sin(p\theta)\\\\ L \frac{di_{b}}{dt} & = & v_{b}-R i_{b}+ p \phi_f \Omega \sin\left(p\theta-\frac{2\pi}{3}\right)\\\\ L \frac{di_{c}}{dt} & = & v_{c}-R i_{c}+ p \phi_f \Omega \sin\left(p\theta+\frac{2\pi}{3}\right)\\\\ J\frac{d\Omega}{dt} &=& \tau_m-\tau_l \\\\ {\frac{d\theta}{dt}} &=& \Omega\\ \end{array}\right. \end{equation}\]

where \(i_{abc} = \left[\begin{matrix}i_a & i_b & i_c\end{matrix}\right]^\intercal\) are the stator phase currents, \(v_{abc} = \left[\begin{matrix}v_a & v_b & v_c\end{matrix}\right]^\intercal\) the stator phase voltages, \(\Omega\) and \(\theta\) are the speed and position respectively and \(p\) the pole pair number. \(L\) and \(R\) and the staotr inductance and resistor, \(\phi_f\) the flux constant. \(\tau_r\) the resistive torque and \(\tau_m\) the motor torque.

\[\begin{equation} \tau_m = -p\phi_f \left[i_a\sin(p\theta) + i_b\sin\left(p\theta-\frac{2\pi}{3}\right) + i_c\sin\left(p\theta+\frac{2\pi}{3}\right)\right] \end{equation}\]

Clarke and Parc transformations

Clarke-Parc Transformations

Clarke transformation : 2-phases equivalent representation

Under the assumption of balanced motor one has \(x_a+x_b+x_c = 0\). Motor equations can thus be transformed into a 2-phases equivalent representation using Clarke transformation.

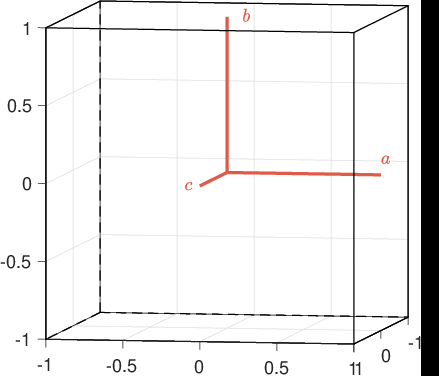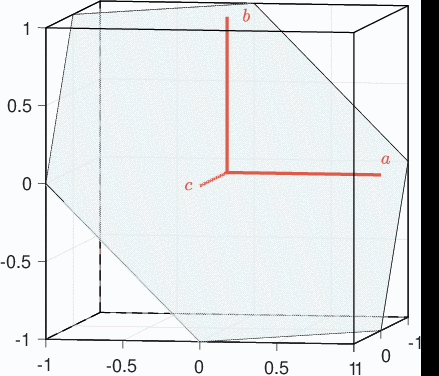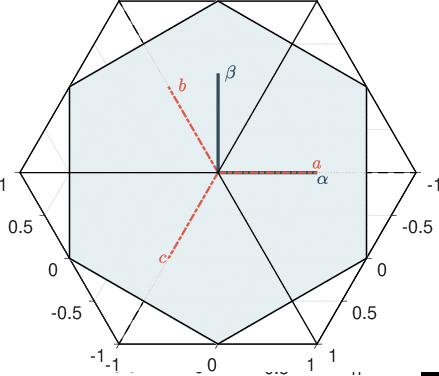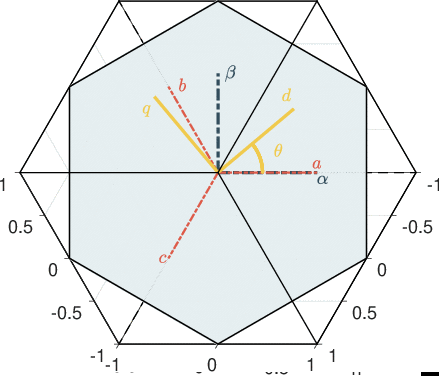
\[\begin{equation} x_{\alpha\beta} = \begin{bmatrix}x_\alpha & x_\beta\end{bmatrix}^\intercal = T_{3\rightarrow2}x_{abc} = T_{3\rightarrow2}\begin{bmatrix}x_a & x_b &x_c\end{bmatrix}^\intercal \end{equation}\] \[\begin{equation} T_{3\rightarrow2} = \frac{2}{3}\begin{bmatrix}1 & -\frac{1}{2}& -\frac{1}{2}\\0 & \frac{\sqrt{3}}{2}& -\frac{\sqrt{3}}{2}\end{bmatrix} \end{equation}\] \[\begin{equation} \label{eq:modeleab} \left\{\begin{array}{rcl} L \frac{di_{\alpha}}{dt} & = & v_{\alpha}-R i_{\alpha}+ p \phi_f \Omega \sin(p\theta)\\\\ L \frac{di_{\beta}}{dt} & = & v_{\beta}-R i_{\alpha}- p \phi_f \Omega \cos\left(p\theta\right)\\\\ J\frac{d\Omega}{dt} &=& \tau_m -\tau_r \\\\ {\frac{d\theta}{dt}} &=& \Omega\\ \end{array}\right. \end{equation}\] \[\begin{equation} \tau_m = p\frac{3}{2}\phi_f \left[-i_\alpha\sin(p\theta) + i_\beta\cos(p\theta)\right] \end{equation}\]

Park transformation : \(d-q\) rotating frame

In the previous model, votages and currents varies at high frequency (p times the rotational frequency). To avoid sinus terms Park transformation is used (Par29).

The Park transform is a rotation matrix \(R(\theta)\) from \(\alpha\beta\) axes to \(dq\) axes. One as

\[\begin{equation} \label{eq:park} x_{dq} = R(\theta) x_{\alpha\beta} = \left[ \begin{matrix} \cos(p\theta) & \sin(p\theta)\\ -\sin(p\theta) & \cos(p\theta) \end{matrix} \right]x_{\alpha\beta} \end{equation}\] \[\begin{equation} \label{eq:invpark} x_{\alpha\beta} = R(\theta)^{-1} x_{dq} =\left[ \begin{matrix} \cos(p\theta) & -\sin(p\theta)\\ \sin(p\theta) & \cos(p\theta) \end{matrix} \right]x_{dq} \end{equation}\]

The model in the \(dq\) frame is :

\[\begin{equation} \label{eq:modeledq} \left\{\begin{array}{rcl} L \frac{di_d}{dt} & = & v_d-R i_d + Lp\Omega i_q\\\\ L \frac{di_q}{dt} & = & v_q-R i_q - Lp\Omega i_d -\phi_f p\Omega\\\\ J\frac{d\Omega}{dt} &=& \tau_m -\tau_r \\\\ {\frac{d\theta}{dt}} &=& \Omega\\ \end{array}\right. \end{equation}\] \[\begin{equation} \tau_m = p\frac{3}{2}\phi_f i_q \end{equation}\]

References :